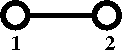The Mayer f-function, or f-bond is defined as:

where
Diagrammatically the Mayer f-function is written as
Hard sphere model
For the hard sphere model the Mayer f-function becomes:

where  is the hard sphere diameter.
is the hard sphere diameter.
References
- Joseph E. Mayer "Contribution to Statistical Mechanics", Journal of Chemical Physics 10 pp. 629-643 (1942)