Fused hard sphere chains: Difference between revisions

Revision as of 11:08, 8 March 2011

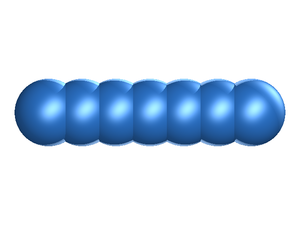
In the fused hard sphere chain model the molecule is built up form a string of overlapping hard sphere sites, each of diameter $\sigma$ .

An effective number of monomers can be applied to the fused hard sphere chain model by using the relarion (Ref. 4 Eq. 2.18)

m_{\rm {effective}}={\frac {[1+(m-1)L^{*}]^{3}}{[1+(m-1)L^{*}(3-L^{*2})/2]^{2}}}

where m is the number of monomer units in the model, and $L^{*}=L/\sigma$ is the reduced bond length.

The volume of the fused hard sphere chain is given by (Ref. 5 Eq. 13)

V_{\rm {FHSC}}={\frac {\pi \sigma ^{3}}{6}}\left({\frac {1}{2}}(m-1)(3L^{*}-L^{*3})+1\right)~~~~L^{*}>0.5

and the surface area is given by (Ref. 5 Eq. 12)

S_{\mathrm {F} HSC}=\pi \sigma ^{2}\left(1+\left(m-1\right)L^{*}\right)

The Vörtler and Nezbeda equation of state is given by

Z_{\mathrm {FHSC} }=1+(1+3\alpha )\eta _{0}(P^{*})+C_{\rm {FHSC}}[\eta _{0}(P^{*})]^{1.83}

where

C_{\rm {FHSC}}=5.66\alpha (1-0.045[\alpha -1]^{1/2}\eta _{0})

and

\eta _{0}(P^{*})={\frac {{\sqrt {1+4(1+3\alpha )P^{*}}}-1}{2+6\alpha }}

Horst L. Vörtler and I. Nezbeda "Volume-explicit equation of state and excess volume of mixing of fused hard sphere fluids", Berichte der Bunsen-Gesellschaft 94 pp. 559- (1990)
Saidu M. Waziri and Esam Z. Hamad "Volume-Explicit Equation of State for Fused Hard Sphere Chain Fluids", Industrial & Engineering Chemistry Research 47 pp. 9658-9662 (2008)

@@ Line 11: / Line 11: @@
 The volume of the fused hard sphere chain is given by (Ref. 5 Eq. 13)
-:<math>V_{\rm FHSC} =\frac{1}{6} \pi \sigma^3 \left( 1 + (m-1)\frac{L^* \left(3-L^{*2}\right)}{2} \right)</math>
+:<math>V_{\rm FHSC} =\frac{\pi \sigma^3}{6}  \left( \frac{1}{2} (m-1)(3L^*  - L^{*3}) +1 \right)  ~~~~ L^* > 0.5 </math>
 and the surface area is given by (Ref. 5 Eq. 12)